The all too familiar, the so-called natural numbers N = {0, 1, 2, …}, somewhat like our everyday conscious experiences, abuse (don’t panic: mathematicians abuse all the time; cf. notation) that very comforting familiarity as a facade. Here’s what’s in store:
Let’s begin with, say, 1 + 1 = 2, which is the arithmetic of abstract sets. If we go leave the universe of sets and go to the category of pointed sets (e.g., Trump as a distinguished person in the set of Americans; I’m trying to get on the nerves of the so-called intellectuals of the USA ;) we find that 1 + 1 = 1. Not unlike the logic that changes with the universe of discourse (admitting a contradiction, say, ‘posina is alive AND posina is not alive’ is nonsense in Boolean logic for it’s the yellow brick road to prove anything and everything is true; however, in geometry, a contradiction, say, Bharat AND Not-Bharat is a legitimate boundary operation to calculate: boundary (Bharat)), number theory does too.
One of the annoying things that Indians have to endure, like it or not, is: our ancestors knew it all, which wouldn’t have bothered me much but for the fact independent India, with its billion+ minds, didn’t contribute and still isn’t contributing to the world of science, which is a shame: particularly shameful, given that my Godavari culture frowns upon people who are broke—so broke with no money to buy even a cup of chai—but shamelessly broadcast how wealthy their great grandfathers were, as in: my grandfather drank ghee, smell my breath if you don’t believe ;) The moral: we failed miserably to build on our profound intellectual inheritance. Here’s my unfailin it all ;)
Consider a couple of numbers: 1969 and 2023. Thanks to the place-value notation we can readily add them. As if exemplifying the difficulty of seeing the visible and / or stating the obvious, place-value notation is a Marxist analysis of number into a dual of dialectical opposites:
number: Places → Values
which is to say that numbers can be construed as functions.
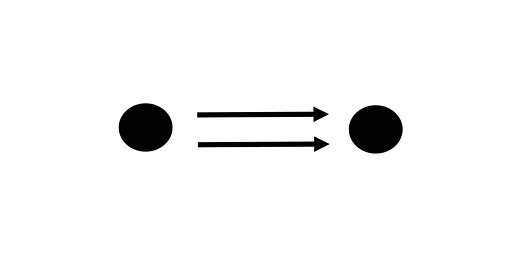
A function
f: A → B
can in turn be construed as a directed graph with one function serving as both source and target functions. In other words, functions are the subcategory of loops (arrows with one dot as both source and target dot) of the category of directed graphs.
Cutting to chase, what good is thinking of numbers as directed graphs?
In characterizing relations between product, sum, and exponentials in various categories of objects, Schanuel noticed algebraic independence of exponentials in the category of directed graphs, which led him to conclude:
Now that we construed numbers as graphs, it seems like we can carry over the above knowledge from graphs to numbers.
P.S. I’ll include additional links little later … (ellipsis is a word and its personal space shouldn’t be violated).
P.P.S. A French postmodernist, when told what he says makes no sense, replied: I’m French.